Dividend Discount Model (DDM): Formula & Examples
The Dividend Discount Model (DDM) is a fundamental quantitative valuation tool used to help determine the intrinsic value of a stock. There are several variations of the model based on future cash flow assumptions of owning a stock.
The goal is to determine a stock’s fair value, then compare it to the market price. If a stock is found to be undervalued via the DDM, then an investor might buy shares. If the formula finds a stock is overvalued compared to the market price, it could be a candidate for a short sale.
The DDM has some shortcomings, and other valuation tools can be used in conjunction with it to help improve the accuracy of your fundamental analysis.
Additionally, traders can combine this fundamental analysis with technical analysis tools to determine optimal entry and exit points when buying and selling shares.
What Is the Dividend Discount Model (DDM)
The DDM uses a discounted cash flow approach to valuing a stock. The idea is that a stock’s value is simply the present value of future dividends when discounted back to the present. This equity valuation technique looks closely at the cash flows of a stock including future dividend payments and the sale of the stock itself at some future date.
You can think of it as a bottom-up investing approach. The dividend discount model is used to find stocks that are either under- or overvalued compared to the market price. Thus, it is used to find long and short ideas using fundamental analysis and equity valuation.
To better understand the DDM, it’s helpful to know how business fundamentals, and fundamental stock analysis, works.
When a firm earns profits, it can either retain those earnings or pay them out as dividends. The DDM can work best with companies that pay out a large proportion of its profits as dividends. The DDM does not work as well on firms that do not distribute dividends or on companies that pay very little out to shareholders.
The dividend discount model formula is also based on the notion of the time value of money, which says that a dollar today is worth more than a dollar in the future. For this reason, firms that have big dividends today are generally thought to be worth more than those that defer them to the future (per the calculation).
💡 Quick Tip: All investments come with some degree of risk — and some are riskier than others. Before investing online, decide on your investment goals and how much risk you want to take.
Dividend Discount Model vs Discounted Cash Flow Model
The Dividend Discount Model (DDM) is closely related to the Discounted Cash Flow Model (DCF) but has distinct differences.
The DDM focuses on the cash flows associated with holding a stock, including dividends and cash received upon a stock sale.
The DCF model examines the cash flows in a company and determines the overall market value of the company. Cash flows include profits, depreciation, changes in accounts receivable, changes in accounts payable, etc. As you might imagine a DCF calculation is extremely detailed and requires some financial and accounting acumen to perform accurately.
Both models require determining future cash flows and forecasting the future requires a mix of art and science to develop accurate valuations.
Dividend Discount Model Formulas
There are several dividend discount model formulas. Each is based on the nature of future dividend distributions from the company to shareholders.
Gordon Growth Model
The Gordon Growth Model (GGM) is one of the most popular versions of the DDM. It is named after American economist Myron Gordon, who first developed the valuation technique. The GGM is also a rather straightforward spin on the DDM since it assumes a stock will pay dividends at a constant rate into perpetuity.
You might use the GGM when analyzing very stable businesses that have steady cash flows and a track record of consistent dividend payouts. Big, blue-chip companies and utility stocks are good examples. The GGM is expressed as:
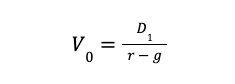
Where:
• V0 = The current stock price
• D1 = The dividend payment one period from now
• r = The required rate of return on the stock
• g = The constant growth rate of the company’s dividends into perpetuity
Be aware that the model is extraordinarily sensitive to the dividend growth rate used.
One-Period Dividend Discount Model
The one-period DDM is used less frequently than the popular Gordon Growth Model. It is useful when an investor wants to calculate a stock’s fair value in order to trade it after one period (often one year). Since it is a one-period look, a single dividend is used along with the proceeds of the sale of the stock. Those are the only two cash inflows.
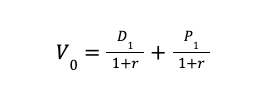
Where:
• V0 = The current stock price
• D1 = The dividend payment one period from now
• P1 = The stock price one period from now
• r = The required rate of return on the stock
Multi-Period Dividend Discount Model
In contrast to the one-period DDM, the multi-period formula assumes that an investor plans to hold a stock over a period that features many dividend payments.
What makes this variation of the DDM tough is that you forecast several future dividends. There is no guarantee that a firm’s payout policy will match your forecast. Like other DDM models, a final return of capital is assumed — the sale price of the stock at the end of the holding period.
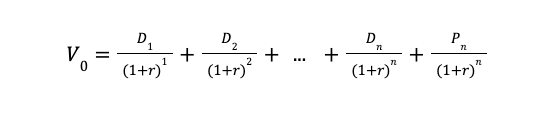
Each future dividend is discounted back to the present using a discount rate that is typically the firm’s estimated cost of equity.
Variable Growth DDM or Non-Constant Growth
You can get even more complex with the variable growth version of the dividend discount model formula. With this approach, you can divide growth into several stages.
Perhaps a firm will grow rapidly over the first year, slow down in year two, then finally transition into a steady grower into perpetuity. Some argue this is a more realistic way to value a stock versus other models. The variable growth DDM assumes non-constant growth by commonly using a two-stage or three-stage approach. Of course, even more stages can be applied.
Zero Growth DDM
A final approach is the zero growth dividend discount model. This is actually the simplest of all DDM variations. It is the same calculation you would use when valuing a perpetuity or preferred stock. It’s simply:
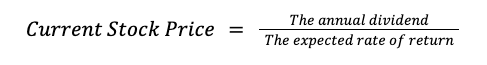
Get up to $1,000 in stock when you fund a new Active Invest account.*
Access stock trading, options, alternative investments, IRAs, and more. Get started in just a few minutes.
Dividend Discount Model Example
Let’s perform an example using the most common DDM method: the Gordon Growth Model.
Suppose a company pays a current annual dividend of $5 (D0) and will grow it at a steady rate of 3% per year into perpetuity. Shares currently trade at $60. We will also assume we used the Capital Asset Pricing Model to find the firm’s 10% estimated cost of equity. Here’s how the DDM would look:
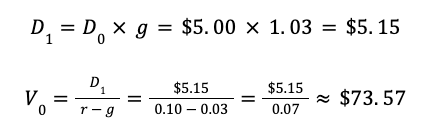
Since we found the stock’s intrinsic value to be significantly higher than the market price, we might buy shares with the thought that eventually the market will realize how valuable the stock is and the price will move towards our valuation.
Interpreting DDM Results
Interpreting the results from the dividend discount model is straightforward, but it is getting to the output that can be tricky. The inputs to the calculation are often subjective and can change over time, so any interpretation should be taken with a grain of salt.
Dividends can be hard to forecast accurately, and valuations are sensitive to the growth and discount rates chosen. The analyst must also be open to the possibility that market forces can cause an over- or under-valued stock to further drift from intrinsic value.
How Investors Can Use DDM
The dividend discount model, and all its variations, can be used to calculate a stock’s fair value. In practice, that fair value is then compared to the market price.
Investors can choose to go long shares when they determine that a company’s intrinsic value is above the market price. They can also short shares if the DDM valuation method determines that a stock is overvalued compared to the market price.
The dividend discount model can be used to value stocks in different sectors to see which might be the best investment.
The use of the DDM is based on fundamental analysis and the notion that stock values ultimately revert to their intrinsic worth based on the present value of future cash flows.
Investors can use the DDM along with other valuation techniques to help form a better mosaic of a company’s value. Moreover, technical analysis indicators could be used for more precise buy and sell price points.
The Takeaway
The dividend discount model formula is one of the most widely used equity valuation techniques. Its premise is that firms pay out a large proportion of their profits as dividends to equity holders, thus an intrinsic value can be calculated using those predictable future cash flows.
There are several variations of the DDM based on the profile of a firm’s future dividends. There are drawbacks to the DDM, and using other valuation methods can help an analyst determine if a stock is over- or under-valued.
Ready to invest in your goals? It’s easy to get started when you open an investment account with SoFi Invest. You can invest in stocks, exchange-traded funds (ETFs), mutual funds, alternative funds, and more. SoFi doesn’t charge commissions, but other fees apply (full fee disclosure here).
Photo credit: iStock/svetikd
SoFi Invest® INVESTMENTS ARE NOT FDIC INSURED • ARE NOT BANK GUARANTEED • MAY LOSE VALUE
1) Automated Investing and advisory services are provided by SoFi Wealth LLC, an SEC-registered investment adviser (“SoFi Wealth“). Brokerage services are provided to SoFi Wealth LLC by SoFi Securities LLC.
2) Active Investing and brokerage services are provided by SoFi Securities LLC, Member FINRA (www.finra.org)/SIPC(www.sipc.org). Clearing and custody of all securities are provided by APEX Clearing Corporation.
For additional disclosures related to the SoFi Invest platforms described above please visit SoFi.com/legal.
Neither the Investment Advisor Representatives of SoFi Wealth, nor the Registered Representatives of SoFi Securities are compensated for the sale of any product or service sold through any SoFi Invest platform.
Financial Tips & Strategies: The tips provided on this website are of a general nature and do not take into account your specific objectives, financial situation, and needs. You should always consider their appropriateness given your own circumstances.
Claw Promotion: Customer must fund their Active Invest account with at least $25 within 30 days of opening the account. Probability of customer receiving $1,000 is 0.028%. See full terms and conditions.
SOIN0221052